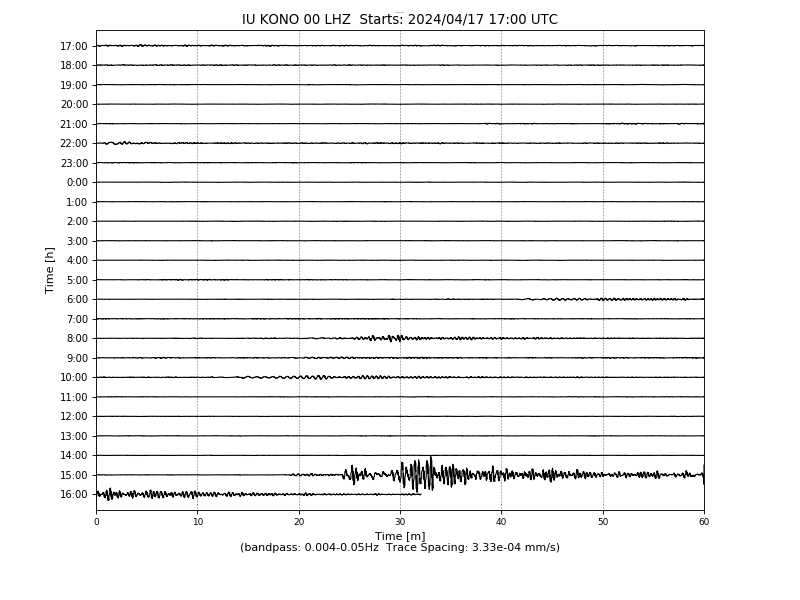
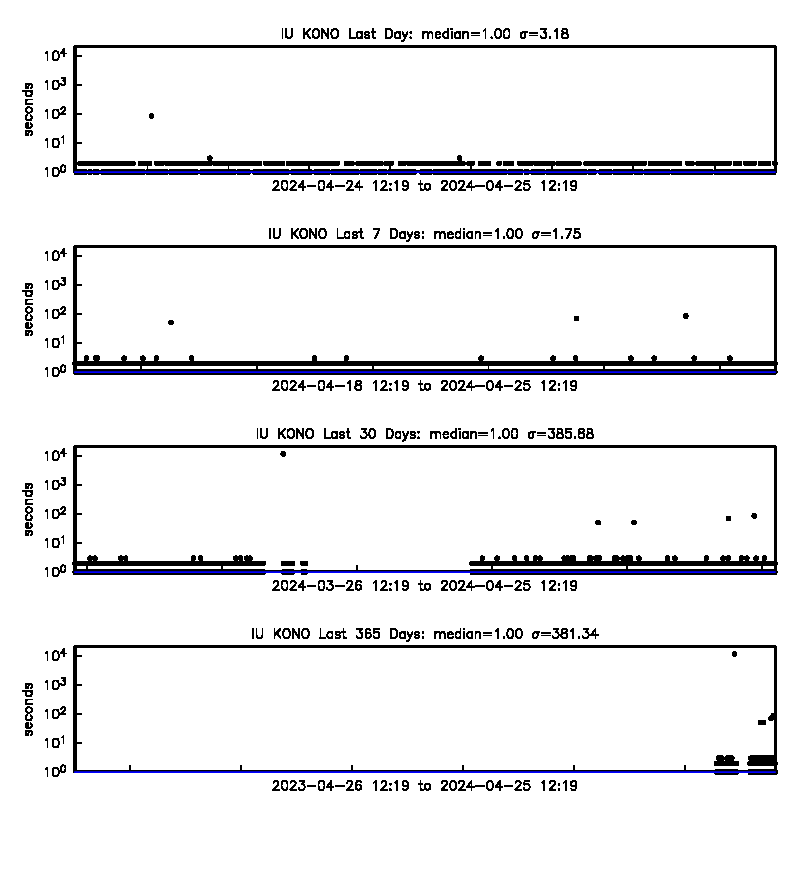
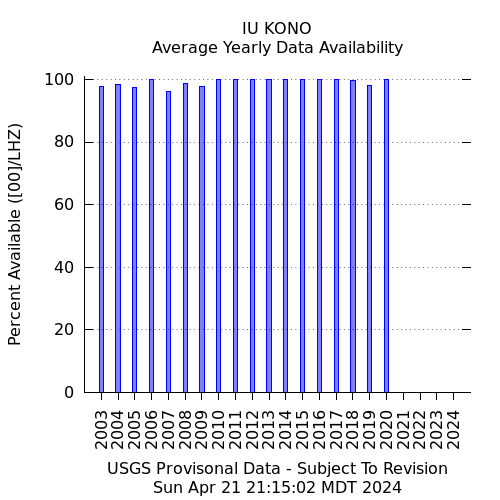
Kongsberg, Norway
IU KONO commences operations on: 1991,171
| Host | University of Bergen |
|---|---|
| Network | IU |
| Latitude | 59.649 |
| Longitude | 9.598 |
| Elevation | 216 |
| Datalogger | Q330-HR |
| Broadband | Streckeisen STS-1VBB w/E300 |
| Accelerometer | Kinemetrics FBA ES-T EpiSensor Accelerometer |
| Telemetry Status at the NEIC | Pending |
 Jump to Navigation
Jump to Navigation